XOR-SAT — различия между версиями
(→Решение XOR-SAT задачи методом Гаусса) |
м (rollbackEdits.php mass rollback) |
||
| (не показано 55 промежуточных версий 5 участников) | |||
| Строка 1: | Строка 1: | ||
{{Задача | {{Задача | ||
| − | |definition = <b><tex>\mathrm {XORSAT}</tex></b> (XOR-satisfiability) выполнимость функции — задача распределения аргументов в булевой [[КНФ|КНФ]] функции, записанной в виде XOR-КНФ, таким образом, чтобы результат данной функции был равен <tex> 1 </tex>. | + | |definition = <b><tex>\mathrm {XORSAT}</tex></b> (англ. ''XOR-satisfiability'') выполнимость функции — задача распределения аргументов в булевой [[КНФ|КНФ]] функции, записанной в виде XOR-КНФ, таким образом, чтобы результат данной функции был равен <tex> 1 </tex>. |
}} | }} | ||
| Строка 6: | Строка 6: | ||
== Описание == | == Описание == | ||
| − | Одним из особых случаев | + | Одним из особых случаев <tex>\mathrm {SAT}</tex> является класс задач, где каждый конъюнкт содержит операции <tex>\oplus</tex> (т. е. исключающее или), а не (обычные) <tex>\lor</tex> операторы.Формально, обобщенная КНФ с тернарным булевым оператором <tex> R</tex> работает только если <tex> 1</tex> или <tex> 3</tex> переменные дают <tex> \mathtt {true}</tex> в своих аргументах. Конъюнкты, имеющие более <tex> 3</tex> переменных могут быть преобразованы в сочетании с формулой преобразования с сохранением выполнимости булевой функции, т. е. <tex>\mathrm {XOR}</tex>-<tex>\mathrm {SAT}</tex> может быть снижена до <tex>\mathrm {XOR}</tex>-<tex>3</tex>-<tex>\mathrm {SAT}</tex><ref>''Alfred V. Aho; John E. Hopcroft; Jeffrey D. Ullman.''The Design and Analysis of Computer Algorithms. Addison-Wesley.; здесь: Thm.10.4, 1974.</ref> |
| + | Это задача [[Класс P|<tex>\mathrm {P}</tex>-класса]], так как <tex>\mathrm {XOR}</tex>-<tex>\mathrm {SAT}</tex> формулу можно рассматривать как систему линейных уравнений по модулю <tex>2</tex>, которая, в свою очередь, может быть решена за <tex>O(n^3)</tex> методом Гаусса <ref>[https://ru.wikipedia.org/wiki/%D0%9C%D0%B5%D1%82%D0%BE%D0%B4_%D0%93%D0%B0%D1%83%D1%81%D1%81%D0%B0 Метод Гаусса]</ref>.Такое представление возможно на основе связи между Булевой алгеброй и Булевым [[Определение кольца, подкольца, изоморфизмы колец|кольцом]] <ref>[https://en.wikipedia.org/wiki/Boolean_algebra_(structure)#Boolean_rings Связь между Булевой алгеброй и Булевым кольцом]</ref> и том факте, что арифметика по модулю <tex>2</tex> образует конечное поле <ref>[https://ru.wikipedia.org/wiki/%D0%9A%D0%BE%D0%BD%D0%B5%D1%87%D0%BD%D0%BE%D0%B5_%D0%BF%D0%BE%D0%BB%D0%B5 Конечное поле ]</ref>. | ||
| − | + | ==Пример решения XORSAT== | |
| − | + | ===Пример=== | |
| − | ==Решение XOR-SAT задачи методом Гаусса= | + | <font color='red'>Красные пункты</font> могут быть добавлены для возможности представления КНФ-функции в виде <tex>\mathrm {XOR}</tex>-<tex>\mathrm {SAT}</tex>. |
| + | {| class="wikitable" | ||
| + | !<tex>(a \oplus b \oplus c) \land (b \oplus \neg c \oplus d) \land (a \oplus b \oplus \neg d) \land (a \oplus \neg b \oplus \neg c)</tex> | ||
| + | ! style="background: #ffdddd;" |<tex> \land (\neg a \oplus b \oplus c) </tex> | ||
| + | |} | ||
| + | {| class="wikitable" | ||
| + | |+ | ||
| + | !colspan="2"|Решение XOR-SAT задачи методом Гаусса | ||
| + | |-align="center" | ||
| + | ! | ||
<center> | <center> | ||
{| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
|+ | |+ | ||
| − | !colspan=" | + | !colspan="2"|Система уравнений |
| − | |||
| − | |||
| − | |||
| − | |||
|-align="center" | |-align="center" | ||
!Переменные | !Переменные | ||
| − | |! width="20%" | | + | |! width="20%" | Значение |
|-align="center" | |-align="center" | ||
! <tex> a </tex> <tex>\oplus</tex> <tex> c </tex> <tex>\oplus</tex> <tex> d </tex> | ! <tex> a </tex> <tex>\oplus</tex> <tex> c </tex> <tex>\oplus</tex> <tex> d </tex> | ||
| Строка 37: | Строка 43: | ||
|-align="center" | |-align="center" | ||
! style="background: #ffdddd;" |<tex> \neg a </tex> <tex>\oplus</tex> <tex> b </tex> <tex>\oplus</tex> <tex> c </tex> | ! style="background: #ffdddd;" |<tex> \neg a </tex> <tex>\oplus</tex> <tex> b </tex> <tex>\oplus</tex> <tex> c </tex> | ||
| − | ! style="background: #ffdddd;" |<tex> \ | + | ! style="background: #ffdddd;" |<tex> =1 </tex> |
| + | |} | ||
| + | </center> | ||
| + | !(«<tex>1</tex>» означает «<tex> \mathtt {true}</tex>», «<tex>0</tex>» означает «<tex> \mathtt {false}</tex>») | ||
| + | Каждый конъюнкт ведет к одному уравнению. | ||
| + | |-align="center" | ||
| + | ! | ||
| + | <center> | ||
| + | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
| + | |+ | ||
| + | !colspan="2"|Нормированная система уравнений | ||
| + | |-align="center" | ||
| + | !Переменные | ||
| + | |! width="20%" | Значение | ||
| + | |-align="center" | ||
| + | ! <tex> a </tex> <tex>\oplus</tex> <tex> c </tex> <tex>\oplus</tex> <tex> d </tex> | ||
| + | |<tex>=1</tex> | ||
| + | |-align="center" | ||
| + | ! <tex> b </tex> <tex>\oplus</tex> <tex> c </tex> <tex>\oplus</tex> <tex> d </tex> | ||
| + | |<tex>=0</tex> | ||
| + | |-align="center" | ||
| + | ! <tex> a </tex> <tex>\oplus</tex> <tex> b </tex> <tex>\oplus</tex> <tex> d </tex> | ||
| + | |<tex>=0</tex> | ||
| + | |-align="center" | ||
| + | ! <tex> a </tex> <tex>\oplus</tex> <tex> b </tex> <tex>\oplus</tex> <tex> c </tex> | ||
| + | |<tex>=1</tex> | ||
| + | |-align="center" | ||
| + | ! style="background: #ffdddd;" |<tex> a </tex> <tex>\oplus</tex> <tex> b </tex> <tex>\oplus</tex> <tex> c </tex> | ||
| + | ! style="background: #ffdddd;" |<tex> =0 </tex> | ||
| + | |} | ||
| + | </center> | ||
| + | !Используя свойства Булевых [[Определение кольца, подкольца, изоморфизмы колец|колец]] | ||
| + | (<tex>\neg x=1 \oplus x</tex>, <tex>x \oplus x=1</tex>),<br> | ||
| + | избавимся от отрицаний в нашей системе | ||
| + | |-align="center" | ||
| + | ! | ||
| + | <center> | ||
| + | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
| + | |+ | ||
| + | !colspan="6"|Матрица соответствующих коэффициентов | ||
| + | |-align="center" | ||
| + | !class="dark"| <tex>a</tex> | ||
| + | !class="dark"| <tex>b</tex> | ||
| + | !class="dark"| <tex>c</tex> | ||
| + | !class="dark"| <tex>d</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| | ||
| + | |Строка | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>A</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>B</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>C</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>D</tex> | ||
| + | |} | ||
| + | </center> | ||
| + | !Составим матрицу по следующему правилу: | ||
| + | Если переменная присутствовала в данном конъюнкте<br> | ||
| + | ставим в ячейку <tex>1</tex>, иначе <tex>0</tex> | ||
| + | |-align="center" | ||
| + | ! | ||
| + | <center> | ||
| + | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
| + | |+ | ||
| + | !colspan="6"|Преобразования, чтобы сформировать | ||
| + | верхнюю треугольную матрицу | ||
| + | |-align="center" | ||
| + | !class="dark"| <tex>a</tex> | ||
| + | !class="dark"| <tex>b</tex> | ||
| + | !class="dark"| <tex>c</tex> | ||
| + | !class="dark"| <tex>d</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| | ||
| + | |Операция | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>A</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>C</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>D</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>B</tex> | ||
| + | |} | ||
| + | </center> | ||
| + | !Поменяем местами строки <tex>B,\ C,\ D</tex>,<br> | ||
| + | чтобы упростить получение верхней треугольной матрицы. | ||
| + | |-align="center" | ||
| + | ! | ||
| + | <center> | ||
| + | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
| + | |+ | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>A</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>E=C \oplus A</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>F=D \oplus A</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>B</tex> | ||
| + | |} | ||
| + | </center> | ||
| + | !Т.к. операция <tex>\oplus</tex> даёт <tex>0</tex> при одинаковых аргументах, | ||
| + | применим её для строк <tex>A,\ C=E</tex> и <tex>A,\ D=F</tex>,<br> | ||
| + | чтобы получить <tex>0</tex> в <tex>1</tex>-м столбце. | ||
| + | |-align="center" | ||
| + | ! | ||
| + | <center> | ||
| + | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
| + | |+ | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>A</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>E</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>G=F \oplus E</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>H=B \oplus E</tex> | ||
| + | |} | ||
| + | </center> | ||
| + | !Теперь применим <tex>\oplus</tex> для строк <tex>E,\ F=G</tex> и <tex>B,\ E=H</tex>,<br> | ||
| + | чтобы получить <tex>0</tex> в <tex>2</tex>-м и <tex>3</tex>-м столбцах. | ||
| + | |-align="center" | ||
| + | ! | ||
| + | <center> | ||
| + | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
| + | |+ | ||
| + | !colspan="6"|Преобразования, чтобы сформировать | ||
| + | диагональную матрицу | ||
| + | |-align="center" | ||
| + | !class="dark"| <tex>a</tex> | ||
| + | !class="dark"| <tex>b</tex> | ||
| + | !class="dark"| <tex>c</tex> | ||
| + | !class="dark"| <tex>d</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| | ||
| + | |Операция | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>I=A \oplus H</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>E</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>J=G \oplus H</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>H</tex> | ||
| + | |} | ||
| + | </center> | ||
| + | !Чтобы получить основную диагональную матрицу,<br> | ||
| + | сделаем <tex>\oplus</tex> <tex>A,\ H=I</tex> и <tex>G,\ H=J</tex>,<br> | ||
| + | чтобы получить <tex>0</tex> в <tex>4</tex>-м столбце выше диагонали. | ||
| + | |-align="center" | ||
| + | ! | ||
| + | <center> | ||
| + | {| class="wikitable" align="center" style="color: blue; background-color:#ffffcc;" cellpadding="10" | ||
| + | |+ | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>K=I \oplus J</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>L=E \oplus J</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>0</tex> | ||
| + | | <tex>J</tex> | ||
| + | |-align="center" | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>0</tex> | ||
| + | !class="dark" style="font-weight:normal"| <tex>1</tex> | ||
| + | !class="green" style="font-weight:normal" style="background: #ddffdd;"| <tex>1</tex> | ||
| + | | <tex>H</tex> | ||
|} | |} | ||
| + | !Осталось сделать <tex>\oplus</tex> <tex>I,\ J=K</tex> и <tex>E,\ J=L</tex>,<br> | ||
| + | потому что они отличаются в <tex>1</tex>-м и <tex>2</tex>-м столбцах. | ||
| + | |-align="center" | ||
</center> | </center> | ||
| + | |} | ||
| + | ===Решение=== | ||
| + | Если <font color='red'>красный пункт</font> присутствует:<i> Решений нет</i><br> | ||
| + | Иначе:<br> | ||
| + | <tex>a=0=\mathtt {false}</tex><br> | ||
| + | <tex>b=1=\mathtt {true}</tex><br> | ||
| + | <tex>c=0=\mathtt {false}</tex><br> | ||
| + | <tex>d=1=\mathtt {true}</tex><br> | ||
==Вычислительная сложность== | ==Вычислительная сложность== | ||
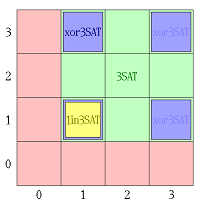
| − | [[Файл:Булева выполнимость.png|400px|thumb|down|Формула с 2-мя дизъюнктами может быть неудовлетворена(красный),< | + | [[Файл:Булева выполнимость.png|400px|thumb|down|Формула с <tex>2</tex>-мя дизъюнктами может быть неудовлетворена(красный), <tex>3</tex>-<tex>\mathrm {SAT}</tex>(зелёный), <tex>\mathrm {XOR}</tex>-<tex>3</tex>-<tex>\mathrm {SAT}</tex>(синий), или/и <tex>1</tex>-<tex>\mathrm {in}</tex>-<tex>3</tex>-<tex>\mathrm {SAT}</tex>, в зависимости от количества переменных со значением <tex> \mathtt {true}</tex> в <tex>1</tex>-м (горизонтальном) и втором (вертикальном) конъюнкте.]] |
| − | Поскольку | + | Поскольку <tex>a \oplus b \oplus c</tex> принимает значение <tex> \mathtt {true}</tex>, если и только если <tex>1</tex> из <tex>3</tex> переменных <tex>\{a,\ b,\ c\}</tex> принимает значение <tex> \mathtt {true}</tex>, каждое решение в <tex>1</tex>-<tex>\mathrm {in}</tex>-<tex>3</tex>-<tex>\mathrm {SAT}</tex> задачи для данной КНФ-формулы является также решением <tex>\mathrm {XOR}</tex>-<tex>3</tex>-<tex>\mathrm {SAT}</tex> задачи, и, в свою очередь, обратное также верно.<br> |
| − | Как следствие, для каждой КНФ-формулы, можно решить | + | Как следствие, для каждой КНФ-формулы, можно решить <tex>\mathrm {XOR}</tex>-<tex>3</tex>-<tex>\mathrm {SAT}</tex>-задачу и на основании результатов сделать вывод, что либо <tex>3</tex>-<tex>\mathrm {SAT}</tex> задача решаема или, что <tex>1</tex>-<tex>\mathrm {in}</tex>-<tex>3</tex>-<tex>\mathrm {SAT}</tex>-задача нерешаема.<br> |
| − | При условии ,что P- и NP-классы не равны,ни 2-,ни Хорн-,ни | + | При условии, что <tex>\mathrm {P}</tex>- и <tex>\mathrm {NP}</tex>-классы не равны, ни <tex>2</tex>-, ни Хорн-, ни <tex>\mathrm {XOR}</tex>-<tex>\mathrm {SAT}</tex> не являются задачи [[Класс NP|<tex>\mathrm {NP}</tex>-класса]], в отличии от <tex>\mathrm {SAT}</tex>. |
== См. также == | == См. также == | ||
| Строка 59: | Строка 356: | ||
*[https://en.wikipedia.org/wiki/Boolean_satisfiability_problem Википедия — Boolean satisfiability problem] | *[https://en.wikipedia.org/wiki/Boolean_satisfiability_problem Википедия — Boolean satisfiability problem] | ||
| − | * Cook, Stephen A. | + | * ''Cook, Stephen A.''Proceedings of the 3rd Annual ACM Symposium on Theory of Computing: 151–158, 1971. |
[[Категория: Дискретная математика и алгоритмы]] | [[Категория: Дискретная математика и алгоритмы]] | ||
| − | [[Категория: Булевы функции ]] | + | [[Категория: Булевы функции]] |
Текущая версия на 19:42, 4 сентября 2022
| Задача: |
| (англ. XOR-satisfiability) выполнимость функции — задача распределения аргументов в булевой КНФ функции, записанной в виде XOR-КНФ, таким образом, чтобы результат данной функции был равен . |
Содержание
Описание
Одним из особых случаев является класс задач, где каждый конъюнкт содержит операции (т. е. исключающее или), а не (обычные) операторы.Формально, обобщенная КНФ с тернарным булевым оператором работает только если или переменные дают в своих аргументах. Конъюнкты, имеющие более переменных могут быть преобразованы в сочетании с формулой преобразования с сохранением выполнимости булевой функции, т. е. - может быть снижена до --[1]
Это задача -класса, так как - формулу можно рассматривать как систему линейных уравнений по модулю , которая, в свою очередь, может быть решена за методом Гаусса [2].Такое представление возможно на основе связи между Булевой алгеброй и Булевым кольцом [3] и том факте, что арифметика по модулю образует конечное поле [4].
Пример решения XORSAT
Пример
Красные пункты могут быть добавлены для возможности представления КНФ-функции в виде -.
| Решение XOR-SAT задачи методом Гаусса | |||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
(«» означает «», «» означает «»)
Каждый конъюнкт ведет к одному уравнению. | ||||||||||||||||||||||||||||||||||||
|
Используя свойства Булевых колец
(, ), | ||||||||||||||||||||||||||||||||||||
|
Составим матрицу по следующему правилу:
Если переменная присутствовала в данном конъюнкте | ||||||||||||||||||||||||||||||||||||
|
Поменяем местами строки , чтобы упростить получение верхней треугольной матрицы. | ||||||||||||||||||||||||||||||||||||
|
|
Т.к. операция даёт при одинаковых аргументах,
применим её для строк и , | ||||||||||||||||||||||||||||||||||||
|
|
Теперь применим для строк и , чтобы получить в -м и -м столбцах. | ||||||||||||||||||||||||||||||||||||
|
Чтобы получить основную диагональную матрицу, сделаем и , | ||||||||||||||||||||||||||||||||||||
|
|
Осталось сделать и , потому что они отличаются в -м и -м столбцах. | ||||||||||||||||||||||||||||||||||||
Решение
Если красный пункт присутствует: Решений нет
Иначе:
Вычислительная сложность
Поскольку принимает значение , если и только если из переменных принимает значение , каждое решение в --- задачи для данной КНФ-формулы является также решением -- задачи, и, в свою очередь, обратное также верно.
Как следствие, для каждой КНФ-формулы, можно решить ---задачу и на основании результатов сделать вывод, что либо - задача решаема или, что ----задача нерешаема.
При условии, что - и -классы не равны, ни -, ни Хорн-, ни - не являются задачи -класса, в отличии от .
См. также
Примечания
- ↑ Alfred V. Aho; John E. Hopcroft; Jeffrey D. Ullman.The Design and Analysis of Computer Algorithms. Addison-Wesley.; здесь: Thm.10.4, 1974.
- ↑ Метод Гаусса
- ↑ Связь между Булевой алгеброй и Булевым кольцом
- ↑ Конечное поле
Источники информации
- Википедия — Boolean satisfiability problem
- Cook, Stephen A.Proceedings of the 3rd Annual ACM Symposium on Theory of Computing: 151–158, 1971.