Деревья Эйлерова обхода

Введение

Динамические деревья (англ.dynamic tree) используются в двух областях:.........

Нужно поддерживать следующие операции

• [math]\mathrm{link(u, w)}[/math] — добавить ребро (u, w) (при условии, что вершины u w принадлежат разным деревьям)
• [math]\mathrm{cut(u, w)}[/math] — разрезать ребро (u, w) (при условии, что ребро (u, w) принадлежит дереву),
• [math]\mathrm{isConnected(u, w)}[/math] — принадлежат ли вершины u и w одной компоненте связности.

Euler tour tree - The data structure we'll develop can perform these operations time O(log n) each.

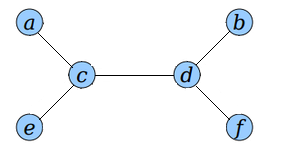
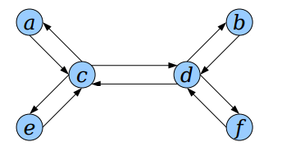
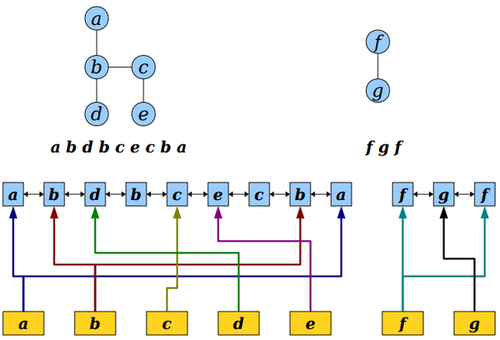
Представление деревьев в виде эйлерова графа

Пример дерева

Соответствующий эйлеров граф

Для представления дерева в виде эйлерового графа заменим каждое ребро [math]\{u, v\} \[/math] дерева на два ребра [math](u, v)[/math] и [math](v, u)[/math].

Получившийся ориентированный граф будет эйлеровым согласно критерию.

Задача:

High-level idea: Instead of storing the trees in the forest, store their Euler tours. Each edge insertion or deletion translates into a set of manipulations on the Euler tours of the trees in the forest. Checking whether two nodes are connected can be done by checking if they're in the same Euler tour.

Свойства эйлерова обхода

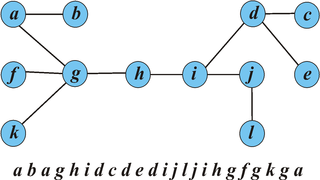
Представим дерево в виде последовательности вершин, посещеннных в порядке эйлерова обхода с корнем в вершине [math]a[/math].

При этом последовательность вершин между первым и последним вхождением вершины [math]h[/math] дает эйлеров обход поддерева с корнем [math]h[/math].

Операции

Изменение корня дерева (переподвешивание)

Для переподвешивания необходимо:

• Разбить эйлеров обход на три части [math]S1 [/math], [math]H[/math], и [math]S2 [/math], где [math]H[/math] состоит из вершин между первым и последним вхождением нового корня [math]h[/math].
• Удалить первую вершину в [math]S1 [/math].
• Соединить в следующем порядке: [math]H[/math], [math]S2 [/math], [math]S1 [/math].
• Добавить [math]\{h\}[/math] в конец последовательности.

В результате получим:

Связывание деревьев

Для связывания деревьев [math]T1 [/math] и [math]T2[/math], где [math]c\in T1\ [/math], а [math]g\in T2\[/math] добавлением ребра [math]\{c, g\} \[/math] необходимо:

• Переподвесить дерево [math]T1[/math] к вершине [math]c[/math].
• Переподвесить дерево [math]T2[/math] к вершине [math]g[/math].
• Соединить получившиеся эйлеровы обходы.
• Добавить [math]\{c\}[/math] в конец последовательности.

В результате получим:

cut(u ,v)

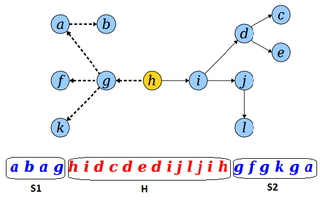
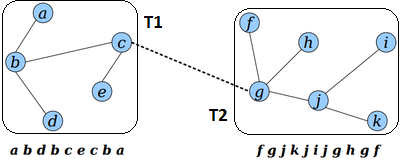
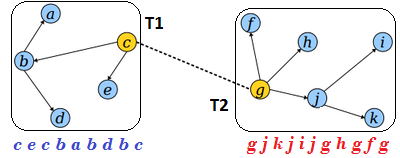
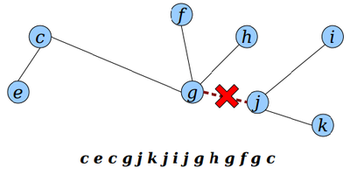
Given a tree T, executing cut(u, v) cuts the edge {u, v} from the tree (assuming it exists).
Watch what happens to the Euler tour of T:

To cut T into T₁ and T₂ by cutting {u, v}:
Let E be an Euler tour for T.
Rotate u to the front of E.
Split E into E₁, V, E₂, where V is the span between the first and last occurrence of v.
T₁ has the Euler tour formed by concatenating E₁ and E₂, deleting the extra u at the join point.
T₂ has Euler tour V.

Реализация структуры

Goal: Implement link, cut, and is-connected as efficiently as possible.

By representing trees via their Euler tours, can implement link and cut so that only O(1) joins and splits are necessary per operation.

Questions to answer:
How do we efficiently implement these joins and splits?
Once we have the tours, how do we answer connectivity queries?

Implementing the Structure

The operations we have seen require us to be able to efficiently do the following:
Identify the first and last copy of a node in a sequence.
Split a sequence at those positions.
Concatenate sequences.
Add a new copy of a node to a sequence.
Delete a duplicate copy of a node from a sequence.
How do we do this efficiently?

Linked Lists

Each split or concatenate takes time O(1).
The first and last copy of a node can be identified in time O(1).
A new copy of a node can be appended to the end of the sequence in time O(1).
A redundant copy of a node can be deleted in time O(1).
Everything sounds great!
Question: How do you test for connectivity?

Euler tours give a simple, flexible encoding of tree structures.
Using doubly-linked lists, concatenation and splits take time O(1) each, but testing connectivity takes time Θ(n) in the worst-case.
Can we do better?

Balanced Trees

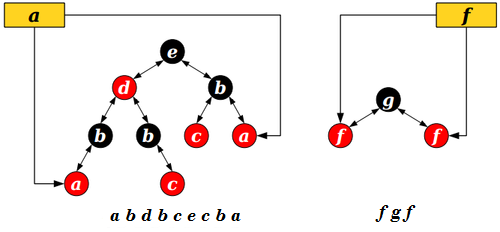
Claim: It is possible to represent sequences of elements balanced binary trees.

These are not binary search trees. We're using the shape of a red/black tree to ensure balance.

Observation 1: Can still store pointers to the first and last occurrence of each tree node.

Observation 2: If nodes store pointers to their parents, can answer is-connected(u, v) in time O(log n) by seeing if u and v are in the same tree.

Observation 3: Red/black trees can be split and joined in time O(log n) each.

The data structure:
Represent each tree as an Euler tour.
Store those sequences as balanced binary trees.
Each node in the original forest stores a pointer to its first and last occurrence.
Each node in the balanced trees stores a pointer to its parent.
link, cut, and is-connected queries take time only O(log n) each.

Сравнительная табличка

Похожие структуры

Про link-cut trees